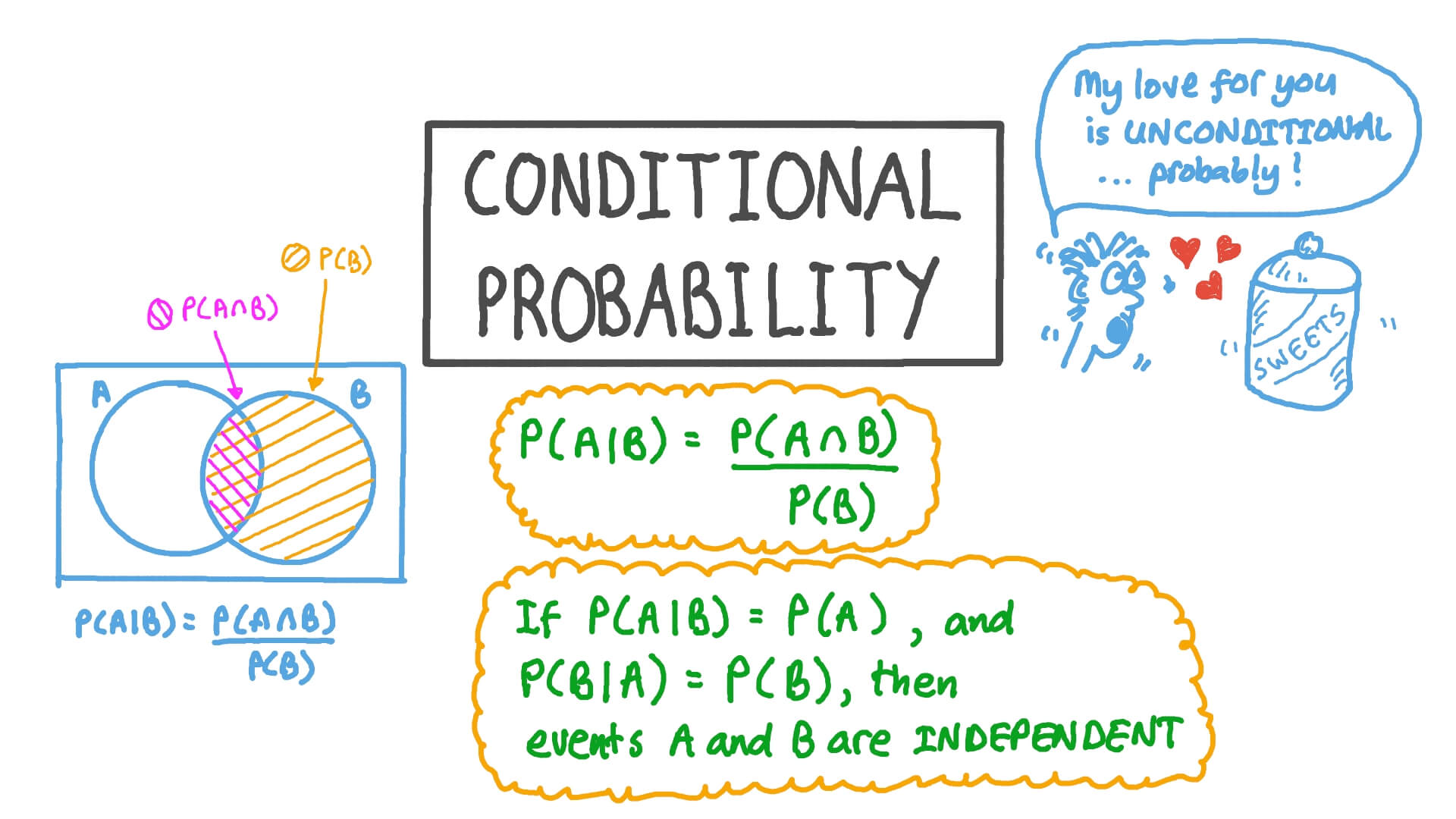
Conditional probability is the measure of the probability of an event or outcome occurring given that another event has already occurred. 

It essentially provides a tool for measuring how likely it is that an event will happen based on the occurrence of some other related event. For example, if we are interested in calculating the probability of it raining tomorrow, we can first look at the probability of it raining today and use this information to estimate what will happen tomorrow.  

Using Bayes’Theorem

In statistics, conditional probability is often represented using Bayes’ theorem, which states that “the probability of an event A given another event B equals the probability of B given A times the unconditional or absolute probability of A divided by the unconditional or absolute probability of B”. 

In other words, Bayes’ theorem can be used to calculate how much more likely one event is over another when taking into consideration any prior knowledge we may have about either events. 

Independent Probabilities 

Another way to think about conditional probabilities is by comparing them to independent probabilities – those which occur without any dependence on other events or occurrences. By definition, independent probabilities are not affected by any external factors, 

Conditional Probabilities

while conditional probabilities can change depending on such things as new evidence emerging or different conditions being present. Understanding conditional probabilities and their relationship with independent ones can provide valuable insight into understanding a wide range of phenomena in both everyday life and more advanced scientific areas such as genetics and medical diagnosis. 

With this knowledge in hand, researchers can make better-informed decisions when predicting future trends and outcomes. Conditional probability is a type of probability that considers an event based on the assumption that one or more other events have already occurred. For example, if Event A has a probability of 0.2, Event B has a probability of 0.3 and Event A and B occur together (the conditional event), then the conditional probability is calculated by dividing the probability of both events occurring together by the probability of Event A occurring alone (i.e. 0.2). 

This would give us a conditional probability for Event B given that Event A has already occurred as 0.6 (i.e. 0.3/0.2). The formula for calculating conditional probability is widely used in many fields such as finance, medicine, and engineering to predict outcomes based on past experiences.

Advantages and Disadvantages

One of the advantages of conditional probability is its ability to provide precise forecasts based on historical data. For example, when a loan officer assesses an individual’s credit score, they can better predict the likelihood of defaulting on a loan by taking into account past credit history. 

Similarly, a medical professional can use a patient’s family history to determine the risk of developing certain diseases, such as cancer. Another advantage of conditional probability is its versatility, as it can be applied to a wide range of problems. 

Its applications extend beyond the fields of finance and medicine and can be used in biology, physics, and many other areas. However, there are also potential disadvantages when using conditional probability. One of these is that it requires a substantial amount of historical data to provide meaningful results. 

Without an adequate sample size, the probability calculation will be unreliable, leading to incorrect predictions. Another disadvantage of conditional probability is the potential for confounding factors. Even if an event has occurred, it may not necessarily be the cause of another event, making it difficult to accurately predict future outcomes. 

Summary

In summary, conditional probability is a useful statistical tool that has many advantages, including its precision and widespread application. However, caution must be exercised when relying on it heavily, as potential disadvantages, such as inadequate sample size and confounding factors, can lead to inaccurate predictions.